Microeconomics - Price Elasticity
Now that you have completed the basics, let us move onto the various learning outcomes on Microeconomics you should look to know for your upcoming exam.
Price Elasticity
In general, the elasticity of a particular variable is the percentage change in quantity demanded or supplied, divided by the percentage change in the variable of concern. This ratio is often called the elasticity coefficient.
Price elasticity is defined as the percentage change in quantity demanded divided by the percentage change in price.
The price elasticity of demand can be expressed as:
Example: Price Elasticity
Where Ep is the price elasticity coefficient, %ΔQ represents the percentage in quantity, and %ΔP represents the percentage in price. If the price of gasoline goes up by 50%, and the quantity demanded decreases by 20%, the price elasticity of gasoline would be:
Ep = %Δ Quantity = -20% = -0.4
%Δ Price +50%
Typically, the negative sign is ignored and we would say that the price elasticity of gasoline is 0.4.
To calculate elasticity we must first have data for quantities purchased at different prices. Suppose that the price of a good goes from P0 to P1, and that we have data for the change in quantity demanded, which goes from Q0 to Q1. The calculation is typically made by dividing the actual change by the average(or midpoint) of the beginning and ending values. Suppose that the quantity demanded of a good goes from 10 to 14. The percentage change in quantity demanded could be expressed as:
(Q0 - Q1) = 4 = 0.333
0.5(Q0 + Q1) 0.5(24)
That number would be multiplied by 100 to get the percentage change, which in this case would be 33.3%.
Similarly, the percentage change in price can be expressed as:
(P0 - P1) x 100
0.5(P0 + P1)
The full elasticity calculation can be simplified by canceling out the 0.5 (one-half) and 100. The more simplified expression can be stated as:
Example:
Suppose, to continue the example given above, that the change in quantity demanded for the good (10 to 14) was in response to a price decrease from $8 to $7. In that case, the elasticity would be expressed as:
(10 - 14) / (10 + 14) = -4 / 24 = -1/6 = -15 = -2.5
(8 - 7) / (8 + 7) 1 / 15 1/15 6
Alternatively, the elasticity could have been calculated as: -4 divided by half of 24, which is equal to -0.333, over 1 divided by half of 15, which equals 0.1333.
So the elasticity would be -0.333 over 0.133 = - 2.5, the same answer as above.
The following definitions apply to calculations of price elasticity:
1) If Ep > 1, Demand is elastic. The percentage change in price will produce a greater percentage in quantity demanded. If the price goes up, then total revenues will go down. If the price goes down, then total revenues willincrease.
2) If Ep < 1, Demand is inelastic. The percentage change in price will produce a lower percentage in quantity demanded. If the price goes up, then total revenues will go up. If the price goes down, then total revenues will decrease. Put simply, these changes will be less drastic than if demand is elastic.
3) If Ep = 1, Demand has unitary elasticity. A percentage in price will produce the exact same percentage change in quantity. Therefore, changes in price will no have effect on total revenues.
If demand is elastic for a product, then a small change in price will cause a large change in quantity demanded. If the demand for a product is inelastic, even a large change in price might cause little change in quantity demanded.
Price Elasticity
In general, the elasticity of a particular variable is the percentage change in quantity demanded or supplied, divided by the percentage change in the variable of concern. This ratio is often called the elasticity coefficient.
Price elasticity is defined as the percentage change in quantity demanded divided by the percentage change in price.
The price elasticity of demand can be expressed as:
| Formula 3.1 |
Example: Price Elasticity
Where Ep is the price elasticity coefficient, %ΔQ represents the percentage in quantity, and %ΔP represents the percentage in price. If the price of gasoline goes up by 50%, and the quantity demanded decreases by 20%, the price elasticity of gasoline would be:
Ep = %Δ Quantity = -20% = -0.4
%Δ Price +50%
Typically, the negative sign is ignored and we would say that the price elasticity of gasoline is 0.4.
To calculate elasticity we must first have data for quantities purchased at different prices. Suppose that the price of a good goes from P0 to P1, and that we have data for the change in quantity demanded, which goes from Q0 to Q1. The calculation is typically made by dividing the actual change by the average(or midpoint) of the beginning and ending values. Suppose that the quantity demanded of a good goes from 10 to 14. The percentage change in quantity demanded could be expressed as:
(Q0 - Q1) = 4 = 0.333
0.5(Q0 + Q1) 0.5(24)
That number would be multiplied by 100 to get the percentage change, which in this case would be 33.3%.
Similarly, the percentage change in price can be expressed as:
(P0 - P1) x 100
0.5(P0 + P1)
| Look Out! Sometimes the denominator used for these percentage change calculations is simply the original value (P0 and Q0). Because the CFA text uses the midpoint method, unless the exam has instructions to the contrary, it would be safer to use the midpoint method. |
The full elasticity calculation can be simplified by canceling out the 0.5 (one-half) and 100. The more simplified expression can be stated as:
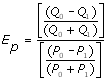 |
Example:
Suppose, to continue the example given above, that the change in quantity demanded for the good (10 to 14) was in response to a price decrease from $8 to $7. In that case, the elasticity would be expressed as:
(10 - 14) / (10 + 14) = -4 / 24 = -1/6 = -15 = -2.5
(8 - 7) / (8 + 7) 1 / 15 1/15 6
Alternatively, the elasticity could have been calculated as: -4 divided by half of 24, which is equal to -0.333, over 1 divided by half of 15, which equals 0.1333.
So the elasticity would be -0.333 over 0.133 = - 2.5, the same answer as above.
The following definitions apply to calculations of price elasticity:
1) If Ep > 1, Demand is elastic. The percentage change in price will produce a greater percentage in quantity demanded. If the price goes up, then total revenues will go down. If the price goes down, then total revenues willincrease.
2) If Ep < 1, Demand is inelastic. The percentage change in price will produce a lower percentage in quantity demanded. If the price goes up, then total revenues will go up. If the price goes down, then total revenues will decrease. Put simply, these changes will be less drastic than if demand is elastic.
3) If Ep = 1, Demand has unitary elasticity. A percentage in price will produce the exact same percentage change in quantity. Therefore, changes in price will no have effect on total revenues.
If demand is elastic for a product, then a small change in price will cause a large change in quantity demanded. If the demand for a product is inelastic, even a large change in price might cause little change in quantity demanded.

No comments:
Post a Comment